published in the journal Sugaku Expositions from American Mathematical Society
Introduction
In the website, we introduce the concept of shape optimization of singular points of a weak solution in boundary value problem of partial differential equations (BVP) defined on a domain $\Omega $, including fracture mechanics, shape optimization of boundary and interface etc. Roughly speaking, singularity is a gap between weak and strong solution (see e.g., [Gr85, Gr92, Kne05, Na94, Sc91]).
We also introduce the integral formula $J_{\omega }(u,\mu )$ named the ``Generalized J-integral'' (GJ-integral) which is the sum of a surface integral $P_{\omega }(u,\mu )$ and a volume integral $R_{\omega }(u,\mu )$ defined on the domain $\omega \cap \Omega $ for an arbitrary domain $\omega $, in which $\omega $ is used to separate the singular points of the solution $u$, and $\mu $ is a vector field representing the perturbation of the singular points.
Generalized J-integral
The GJ-integral is proposed by the author when studying 3-dimensional fracture phenomena [Oh81] as an extension of the J-integral [Ch67, Ri68, Ri68-2] in fracture mechanics (in 2-dimensional).
There are two important properties of the J-integral:- J-1
- The J-integral is equal to energy release rate (ERR) which is an important parameter in fracture mechanics.
- J-2
- The J-integral depends only on the coefficient of the singular term at the crack tip called the stress intensity factor (SIF) (see e.g., [Bu04, Ri68-2]).
The GJ-integral is defined in various BVPs and applicable to such shape sensitivity of boundary [Oh85] not only fracture mechanics [Oh81].
There are three important properties of the GJ-integral:- GJ-1
- Independence from boundary conditions. The GJ-integral is made from partial differential equations only (see the proof of Theorem 2.2 ).
- GJ-2
- The GJ-integral takes zero if the solution is regular, that is, $J_{\omega }(u,\mu )=0$ for all vector field $\mu $ if $u$ is regular inside $\omega \cap \Omega $, which corresponds to (J-2).
- GJ-3
- The shape sensitivity of energy is almost equal to $-R_{\Omega }(u,\mu )$ which is well defined for the weak solution $u$ (see Corollary 2.13 ), corresponding to (J-1).
A simple example of the use of these properties is the following: If $u$ is regular inside $\Omega $, then from (GJ-3) and (GJ-2) the shape sensitivity of energy is almost equal to $P_{\Omega }(u,\mu )$, that is, the boundary expression is well obtained by shape optimization (see e.g., [Al07, N-S13, Sok92]).
The idea of separating singularity came from [Es56] and originated from the conservation laws (derived from Noether's theorem [Noe18]). A generalization of the Hadamard variational formula [F-O78, G-S52, Had68] is given in Theorem 3.1 and here is called the GJ-Hadamard formula. (GJ-3) is led using the two methods:
The first method is applicable if the material derivative become admissible function (Theorem 2.8 ). The second method is that sensitivity analysis at a minimizer of functional is expressed only by the shape derivative (Theorem 2.10 ), and is available in the nonlinear case. In the Stokes problem, due to the limitation of $\textrm{div}u=0$, it is difficult to use the mapping technique .
This problem can be solved partly by using the Lagrangian multiplier method (Section 2.4 ).
Optimization
The second stage of shape optimization is how to search for the optimal shape using shape sensitivity analysis. Searching methods are classified as parametric or nonparametric. In the parametric method, the searching problem is reduced to a finite dimensional optimization problem [Sa99] which is defined by so-called control points on the boudnary or diameter, length, or thickness, etc.
On the other hand, in nonparametric shape optimization problems, a displacement vector denoting the domain variation from an initial domain to a new domain is selected as the design variable. Because the displacement vector is a function, the nonparametric shape optimization problem becomes a functional optimization problem. In this problem, the number of design parameters is infinite. Thus, we can expect a finer result than that for parametric optimization problems.
Mohammadi and Pironneau proposed a method of remaking a smooth boundary by using the Laplace operator on the boundary [M-P01], which was recently used in [Al07]. Azegami has developed the gradient method in the Hilbert space $H^1(\Omega ;\mathbb{R}^d)$ and called this method the traction method [Az94, A-W96], and later changed the name to the H1-gradient method [Az20]. In this method, domain variation is obtained as a solution to a boundary value problem of an elliptic partial differential equation, such as a linear elastic problem defined in the current domain (see [Az17, Az20] for applications). Since these methods are mathematically equivalent, they are collectively referred to as the ``H1-gradient method'' in this monograph, which is suitable for an optimal shape search using the GJ-integral.
Numerical analysis
The last stage is numerical analysis. Here, the finite element method (FEM) is used for numerical analysis. The stability of the H1-gradient method using the GJ-integral [Oh12, Oh14, Oh17, Oh18] by FEM is shown in Section 3.4 , and the calculation procedure for energy, mean compliance, least mean-square error, eigenvalue as a cost function is shown in Section 3.5 .
Unfortunately, the H1-gradient method is not suitable for crack growth as shown in Section 3.3 .
In actual calculation, it is necessary to create more optimal shapes (moving mesh) from the domain where calculation starts, mesh adaptation to prevent collapse of the mesh by moving mesh, and so on. Here we use FreeFem++ [A-P06, ffempp, Oh14] which can be realized by a mathematical description developed by O.Pironneau and F.Hecht of Laboratoire Jacques-Louis Lions in Paris 6, for the calculation of the test problems in Section 4 .
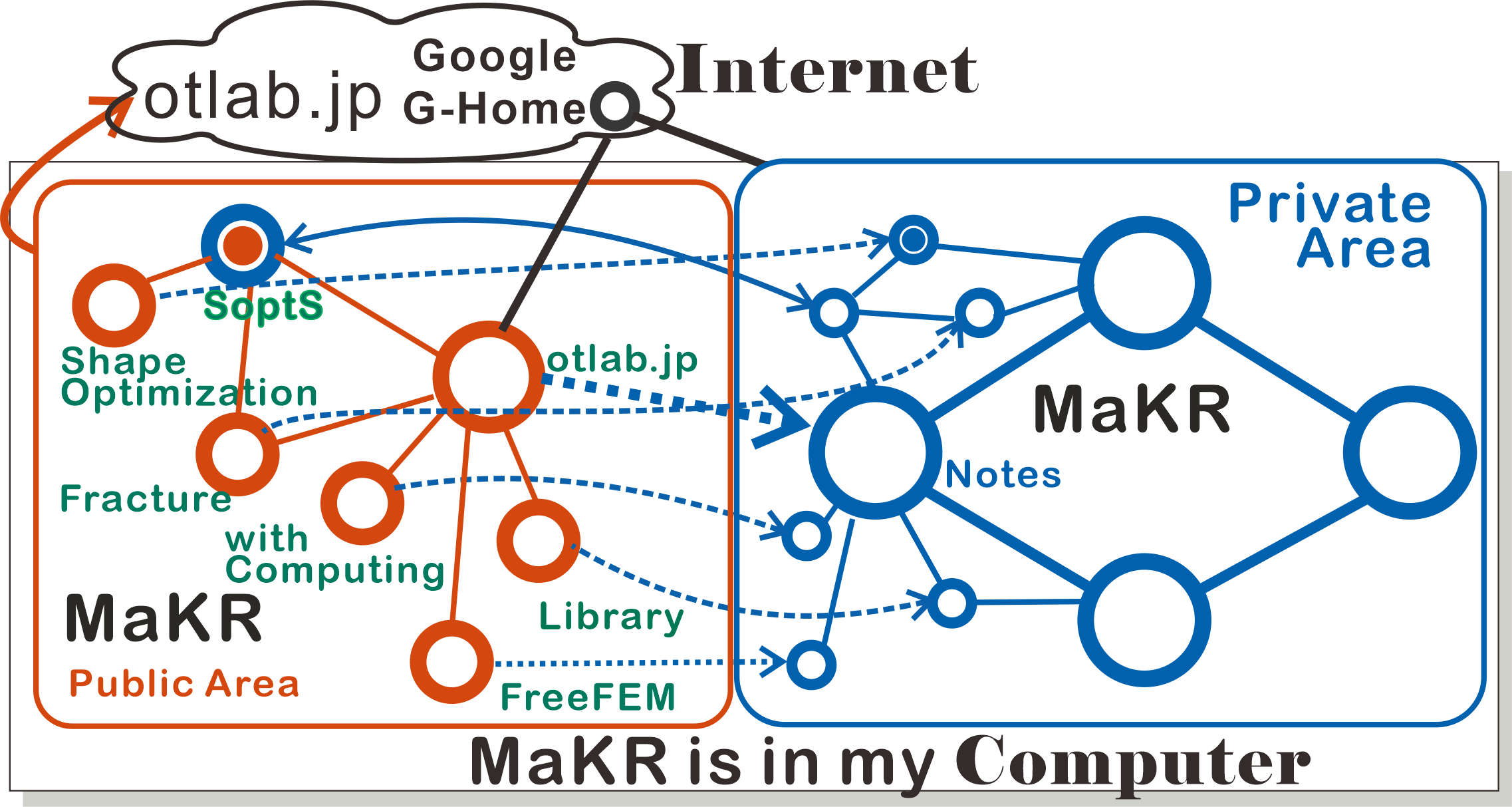
Information about the page: The current position is painted circle in the diagram below. Blue is the main MaKR and orange is a duplicate for MaKR's public use, where dashed line means the connection to the private area The dashed lines are only connections to main MaKR.