Shape sensitivity of energy
Energy shape sensitivity of singular points
We now obtain the energy shape sensitivity of (2.1) as a generalization of Theorem 2.7 . Assume that the variational problem (2.1) is perturbed using a maps $[t\mapsto \varphi _t]\in C^1([0,T);W^{1,\infty }(\mathbb{R}^d;\mathbb{R}^d))$ satisfying $\varphi _0(x)=x$. Here we assume that $\varphi _t$ is bijective from $\Omega $ onto $\Omega (t)=\varphi _t(\Omega )$, then $[v\mapsto v\circ \varphi _t^{-1}]$ is bijection from $H^1(\Omega ;\mathbb{R}^m)$ onto $H^1(\Omega (t);\mathbb{R}^m)$. We now define the perturbation of $V(\Omega )$ by \begin{equation} V^{\varphi _t}(\Omega (t)) :=\{v\circ \varphi _t^{-1}:\, v\in V(\Omega )\} \tag{2.24} \end{equation} Perturbed problem of (2.1) : For given functions $f,g\in H^1(\mathbb{R}^d;\mathbb{R}^m)$ assuming that $\varphi _t(\textrm{supp}_{\Gamma _N}g)=\textrm{supp}_{\Gamma _N}g$ for all $t$, find a minimizer $u^{\varphi _t}$ over $V^{\varphi _t}(\Omega (t))$, that is, \begin{eqnarray} \mathcal{E}(u^{\varphi _t};f,g,\Omega (t))&=&\min _{w\in V^{\varphi _t}(\Omega (t))}\mathcal{E}(w;f,g,\Omega (t)) \tag{2.25a} \end{eqnarray} \begin{eqnarray} \mathcal{E}(w;f,g,\Omega (t))&:=&\int _{\Omega (t)}\{\widehat{W}(w)-fw\} dx- \int _{\Gamma _N}gw\, ds \tag{2.25b} \end{eqnarray} If $V(\Omega )=\{v:\, v=0 \textrm{on }\Gamma _D\}$, then \begin{eqnarray} V^{\varphi _t}(\Omega (t))&=&\{w\in H^1(\Omega (t);\mathbb{R}^m):\, w=0 \textrm{on }\Gamma _D(t)\} , \tag{2.26}\\ &&~~~~~~~~~~\Gamma _D(t)=\varphi _t(\Gamma _D)\notag \end{eqnarray} However, if $V(\Omega )=\{v\in H^1_0(\Omega ;\mathbb{R}^d):\, \textrm{div}v=0\textrm{ in }\Omega \}$, then $$ V^{\varphi _t}(\Omega (t))\neq \{w\in H^1_0(\Omega (t);\mathbb{R}^d):\, \textrm{div}w=0 \textrm{in }\Omega (t)\}$$ The mapping $[v\mapsto v\circ \varphi _t^{-1}]$ become 1-1 mapping from $V(\Omega )$ onto $V^{\varphi _t}(\Omega (t))$. Substituting $v^t=v\circ \varphi ^{-1}$ to $w$ in the right-hand side of (2.25b) , we obtain the following \begin{eqnarray*} \mathcal{E}(v^t;f,g,\Omega (t))&=& \tilde{\mathcal{E}}(v;f,g,\varphi _t)\\ \tilde{\mathcal{E}}(v;f,g,\varphi _t) &:=&\int _{\Omega }\left \lbrace \widehat{W}(\varphi _t(x),v,\Lambda (\varphi _t)\nabla v)-(f\circ \varphi _t)v\right \} \textrm{det}\nabla \varphi _tdx-\int _{\Gamma _N}gv\,ds\\ \Lambda (\varphi )&=&\left (\nabla \varphi ^T\right )^{-1} \in L^\infty (\mathbb{R}^{d},\mathbb{R}^{d\times d}), \textrm{det}\nabla \varphi _t\in L^\infty (\mathbb{R}^d) \end{eqnarray*} The assumption $\varphi _t(\textrm{supp}_{\Gamma _N}g)=\textrm{supp}_{\Gamma _N}g$ means that the part $\{x\in \Gamma _N:g(x)\neq 0\}$ is fixed. The part $\{x\in \Gamma _N:\, g(x)=0\}$ can be freely perturbed. For the solution $u$ of the problem (2.1) , we have \begin{eqnarray} \frac{d}{dt}\mathcal{E}(u^t;f,g,\Omega (t))|_{t=0}&=& \frac{d}{dt}\tilde{\mathcal{E}}(u;f,g,\varphi _t)|_{t=0}\notag \\ &=&-R_{\Omega }(u,\mu _{\varphi })\notag \\ &&-\int _{\partial \Omega }fu(\mu _{\varphi }\cdot n)ds \tag{2.27} \\ &&~~~~~~ \mu _{\varphi }=d\varphi _t/dt |_{t=0}\notag \end{eqnarray} Here we used that for all $\mu \in W^{1,\infty }(\mathbb{R}^d;\mathbb{R}^d)$ $$ \frac{d}{dt}\Lambda (x+t\mu )|_{t=0}=-\Lambda (x)\nabla \mu ^T=-\nabla \mu ^T$$ because $\Lambda (x+t\mu )(I+t\nabla \mu ^T)=I$ ($I$: identity matrix of degree $d$). The right-hand side of (2.27) gives the partial derivative $\partial _{\varphi }$ of $\tilde{\mathcal{E}}(u;f,g,\varphi )$ with respect to $\varphi $.Linear problem
If the problem (2.1)
is linear, i.e.,
\begin{equation} a(u,v;\Omega )=\int _{\Omega }fv\, dx+\int _{\Gamma _N}gv\, ds ∀v\in V(\Omega ) \tag{2.28}
\end{equation} where $a(v,w;\Omega )=\int _{\Omega }\delta \widehat{W}(v)[w]dx$ is a continuous bilinear form on $V:=V(\Omega )$, and $V$ is a subspace of the Hilbert space $X:=H^1(\Omega ;\mathbb{R}^m)$. Here we notice that $a(u,u;\Omega )=2\int _{\Omega }\widehat{W}(u)dx$. Assume the coecivity of $a$, i.e., $$ a(v;\varphi _0)\ge \alpha \|v\|_X^2, ~~\textrm{for all }v\in V$$ with a positive constant $\alpha $, where $a(v;\varphi _0)=a(v,v;\Omega )$. The partial derivative $\partial _\varphi a(v;\varphi _0)[\mu ]=\lim _{\epsilon →0}\epsilon ^{-1}[a(v;\varphi _0+\epsilon \mu )-a(v;\varphi _0)]$ is written as $$ 2\int _{\Omega }\left \{ \nabla _{\xi }\widehat{W}(v)\cdot \mu -\nabla _{\zeta }\widehat{W}(v):[\nabla v\nabla \mu ]+\widehat{W}(v)\,\textrm{div}\mu \right \} \,dx$$ when $\varphi _0(x)=x$ from (2.27)
. $\partial _\varphi a(v;\varphi _0)$ satisfy the following condition
\begin{equation} |\partial _\varphi a(v;\varphi _0)[\mu ]|\le e_1(\varphi _0)\|\mu \|_M \|v\|_X^2 ~~\textrm{for all small }\|\mu \|_M \tag{2.29}
\end{equation} with $M:=W^{1,\infty }(\Omega ;\mathbb{R}^m)$ where $e_1(\varphi _0)$ is a constant depend on $\varphi _0$. From the perturbation theory [Lemma VI.3.1, Kato], there exists a bounded operator $C_1(\varphi _0,\mu )$ on $L^2(\Omega ;\mathbb{R}^m)$ to $L^2(\Omega ;\mathbb{R}^m)$ such that $\partial _\varphi a(u;\varphi _0)[\mu ] =(C_1(\varphi _0,\mu )A_{\varphi _0}^{1/2}u,A_{\varphi _0}^{1/2}u)_{\Omega }$ where $[u\mapsto A_{\varphi }u]$ is defined by $$ (A_{\varphi }u,v)_{\Omega }:=a(u,v;\varphi ) \textrm{for all }v\in V$$
[3.5.4, Hau86]
Theorem 2.8
Let $a^r(v;\mu )$ be the remainder term with the property
\begin{eqnarray} a^r(v;\mu )&:=&a(v;\varphi _0+\mu )-a(v;\varphi _0)-\partial _\varphi a(v;\mu ), \notag \\ |a^r(v;\mu )|&\le & e_2(\varphi _0,\mu )\|\mu \|_M \|v\|_X^2, \tag{2.30}
\end{eqnarray} where $e_2(\varphi _0,\mu )→0$ as $\|\mu \|_M→0$. Then there is the Fréchet derivative $D_{\varphi }A^{-1}_{\varphi _0}$ of $A^{-1}_{\varphi _0}$ with respect to $\varphi $ exists, and $D_\varphi A^{-1}_{\varphi _0}$ is given as
\begin{equation} D_{\varphi }A^{-1}_{\varphi _0}=-(A_{\varphi _0}^{1/2})^{-1}C_1(\varphi _0,\mu ) (A_{\varphi _0}^{1/2})^{-1}. \tag{2.31}
\end{equation} The material derivative $\dot{u}^{\varphi }:=\lim _{t→0}t^{-1}(u^{\varphi _t}\circ \varphi _t-u)$ is given by
\begin{equation} \dot{u}^{\varphi }=-(A_{\varphi _0}^{1/2})^{-1}C_1(\varphi _0,\mu ) (A_{\varphi _0}^{1/2})^{-1}f+ A_{\varphi _0}^{-1}(\nabla f\cdot \mu +f\textrm{div}\mu ) \tag{2.32}
\end{equation} which means that $\dot{u}^{\varphi }\in V$ if $f\in H^1(\Omega ;\mathbb{R}^m)$. Notes
Eq. (3.5.36) in [p289, Hau85] is Eq. (2.32). In [Theorem 3.5.1,p287, Hau85], Eq.(2.30) is proved for Poisson Eq. [Lemma.3.5.2,p185, Hau85]. In [p287, Hau85], it states that (2.30) holds for BiHarmonic (Kirchhoff plate,p184), Linear Elasticity (p186) and Interface problem of linear elasticity (p187) in the same way. As far as the proof are checked, the regularity of $u$ will not need.
Since $\dot{u}^{\varphi }$ is in $V$, we have by $u^{\varphi _t}=u^t+t\dot{u}^{\varphi }\circ \varphi _t^{-1}+o(t)$
\begin{eqnarray} \frac{d}{dt}\mathcal{E}(u^{\varphi _t};f,g,\Omega (t))|_{t=0}&=& \delta _X\mathcal{E}(u;f,g,\Omega )[\dot{u}^{\varphi _t}]+ \partial _{\varphi }\tilde{\mathcal{E}}(u;f,g,\varphi ), \tag{2.33} \\ \delta _X\mathcal{E}(u;f,g,\Omega )[v]&:=& \lim _{\epsilon →0}\epsilon ^{-1}[\mathcal{E}(u+\epsilon v;f,g,\Omega )-\mathcal{E}(u;f,g,\Omega )].\notag
\end{eqnarray}
Main theorem
The function $[t\mapsto \mathcal{E}(u+t\dot{u}^{\varphi };f,g,\Omega )]$ takes the minimum at $t=0$, so that $\delta _X\mathcal{E}(u;f,g,\Omega )[\dot{u}^\varphi ]=0$.
Corollary 2.9
Under the same assumption in Theorem 2.8
, if $\varphi _t(\textrm{supp}_{\Gamma _N}g)=\textrm{supp}_{\Gamma _N}g$ for all $t$, we have the energy shape sensitivity
\begin{eqnarray} \frac{d}{dt}\mathcal{E}(u^{\varphi _t};f,g,\Omega (t))|_{t=0}&=& -R_{\Omega }(u,\mu _{\varphi })\notag \\ &&-\int _{\partial \Omega }fu(\mu _{\varphi }\cdot n)ds,\tag{2.34}\\ \mu _{\varphi }&=&\frac{d}{dt}\varphi _t |_{t=0}.\notag
\end{eqnarray} Notes Without showing $\dot{u}^{\varphi }\in V(\Omega )$, we can prove that (2.34) holds in an abstract framework, which is useful for nonlinear problems and when the solution has singularity. Let $\mathcal{E}(v;\varphi )$ be a functional defined on $\mathcal{V}\times \mathcal{O}$ where $\mathcal{V}\subset X, \mathcal{O}\subset M$ with real Banach spaces $X, M$. Find a minimizer $u(\varphi )\in \mathcal{V}$ for $\varphi \in \mathcal{O}$ such that $$ \mathcal{E}(u(\varphi );\varphi )=\min _{v\in \mathcal{V}}\mathcal{E}(v;\varphi )$$ By $\delta _X, \partial _\varphi $, we denote the partial derivative with respect to $X$ and $M$, repectively. We now assume the following.
- (F1)
- $[\varphi \mapsto \mathcal{E}(v;\varphi )]\in C^{1}(\mathcal{O})$ for all $v\in \mathcal{V}$, and $\partial _{\varphi }\mathcal{E}:~\mathcal{V}\times \mathcal{O}→M^{'}$ is continuous at $(u(\varphi _{0}) ,\varphi _{0})$, where $M'$ is the dual space of $M$.
- (F2)
- The Banach space $X$ is reflexive and $\mathcal{V}$ is closed and convex in $X$.
- (F3)
- For the functional $[v\mapsto \mathcal{E}(v,\varphi _0)]$, $u(\varphi _0)$ is a unique minimizer over $\mathcal{V}$.
- (F4)
- The functional $[v\mapsto \mathcal{E}(v,\varphi _0)]$ is sequentially lower semicontinuous with respect to the weak topology of $X$.
- (F5)
- There is a monotone nondecreasing function $\beta _{0}$ defined on $[0,\infty )$ with $\lim _{s→\infty } \beta _{0}(s)=\infty $ such that $$ \beta _{0}\left ( \left \Vert v\right \Vert _{X}\right ) \leq \mathcal{E}\left ( v,\varphi \right )~~~~~(v\in \mathcal{V},~\varphi \in \mathcal{O}).$$
- (F6)
- For any $\varepsilon \gt 0$ and $R \gt 0$, there exists $\delta \gt 0$ such that \begin{eqnarray*} &&\left | \mathcal{E}( v,\varphi ) -\mathcal{E}(v,\varphi _{0}) \right | \leq \varepsilon \\ &&~~(v\in V,~ \| v\|_X\leq R, ~~\varphi \in \mathcal{O},~\|\varphi -\varphi _0\|_M\leq \delta ). \end{eqnarray*}
- (F7)
- For $v\in V$, the function $[\epsilon \mapsto \mathcal{E}(u(\varphi _0)+\epsilon (v-u(\varphi _0));\varphi _0)]$ belongs to $C^1((0,1])$. Moreover, for a sequence $\{ u_n\} _n\subset \mathcal{V}$ which weakly converges to $u(\varphi _0)$ as $n→\infty $, the condition $\overline{\lim }_{n→\infty } \delta _{X}\mathcal{E}( u_{n},\varphi _{0})[u_{n}-u(\varphi _0)]\leq 0$ implies that $u_{n}→u(\varphi _0)$ strongly in $X$ as $n→\infty $.
Theorem 2.10
[Theorem 3, OT-K12] Under the conditions \textrm{(F1)--(F7)}, $[\varphi \mapsto \mathcal{E}(u(\varphi );\varphi )]$ is Fréchet differentiable at $\varphi =\varphi _{0}$ and the following holds;
\begin{equation} D_{\varphi }\mathcal{E}(u(\varphi _0),\varphi _0)[\mu ]=\partial _{\varphi }\mathcal{E}(u(\varphi _{0}),\varphi _{0})[\mu ] \tag{2.35}
\end{equation} where $D_{\varphi }$ denotes the Fréchet differential operator. Remark 2.11
In [K-W06, K-W11], ((2.35)
) has been proved for semilinear problems.The existence of G\^{a}teaux differentiable at the saddle point in the min-max problem is shown in [Co85], and similar results as Theorem 2.10
also hold. There are many applications to shape optimization (see e.g.[D-Z88, St14]). Remark 2.12 S+ condition
Let $V$ be a closed subspace of $W^{1,p}(\Omega )$ such that $W^{1,p}_0(\Omega )\subset V\subset W^{1,p}(\Omega )$. Then $p$-Laplacian has the condition (F7), where $\widehat{W}(v)=|\nabla v |^p/p$ for $1 \lt p \lt \infty $ ($m=1$) (see e.g. [OT-K12]). $\mathcal{E}(v,\varphi _0)$ is called uniformly monotone iff $$ \{\delta _X\mathcal{E}(v,\varphi _0)-\delta _X\mathcal{E}(w,\varphi _0)\} [v-w] \ge \beta (\|v-w\|_X)\|v-w\|_X$$ for all $v,w\in V$, where the continuous function $\beta :\mathbb{R}_+→\mathbb{R}_+$ is strictly monotone increasing with $\beta (0)=0$ and $\beta (t)→\infty $ as $t→\infty $. If $\mathcal{E}(v,\varphi _0)$ is uniformly monotone, then (F7) holds (see e.g., [Example 27.2(b), Zei/2B]), Corollary 2.13
If , for $\varphi $ near $\varphi _0$ in $C^{0,1}(\Omega _0;\mathbb{R}^d)$, $\varphi _0(x)=x, \overline{\Omega }\subset \Omega _0$,
\begin{eqnarray} \tilde{\mathcal{E}}(v;f,g,\varphi )&:=& \int _{\Omega }\Big \lbrace \widehat{W}(\varphi (x),v,(\nabla \varphi ^T)^{-1}\nabla v)\notag \\ &&~~~~~~~~~~~~-(f\circ \varphi )v\Big \} \textrm{det}\nabla \varphi dx \tag{2.36}\\ &&-\int _{\Gamma _N}gv\,ds\notag
\end{eqnarray} satisfies the conditions (F1)--(F7), then (2.34)
\begin{eqnarray} \frac{d}{dt}\mathcal{E}(u^{\varphi _t};f,g,\Omega (t))|_{t=0}&=& -R_{\Omega }(u,\mu _{\varphi })\notag \\ &&-\int _{\partial \Omega }fu(\mu _{\varphi }\cdot n)ds,\tag{2.34}
\end{eqnarray} holds when $\varphi _t=\varphi _0+t\mu _{\varphi }$ and $\varphi _t(\textrm{supp}_{\Gamma _N}g)=\textrm{supp}_{\Gamma _N}g, 0\le t \lt \epsilon $ with sufficiently small $\epsilon \gt 0$. Corollary 2.14
In addition to the hypotheses of Corollary 2.13
, let us assume that $u$ has the interior regularity, and is in $H^s(\Omega ;\mathbb{R}^m), s \gt 3/2$. Then
\begin{eqnarray} \frac{d}{dt}\mathcal{E}(u^{\varphi _t};f,g,\Omega (t))|_{t=0}&=& P_{\Omega }(u,\mu _{\varphi })-\int _{\partial \Omega }fu(\mu _{\varphi }\cdot n)ds \tag{2.37}
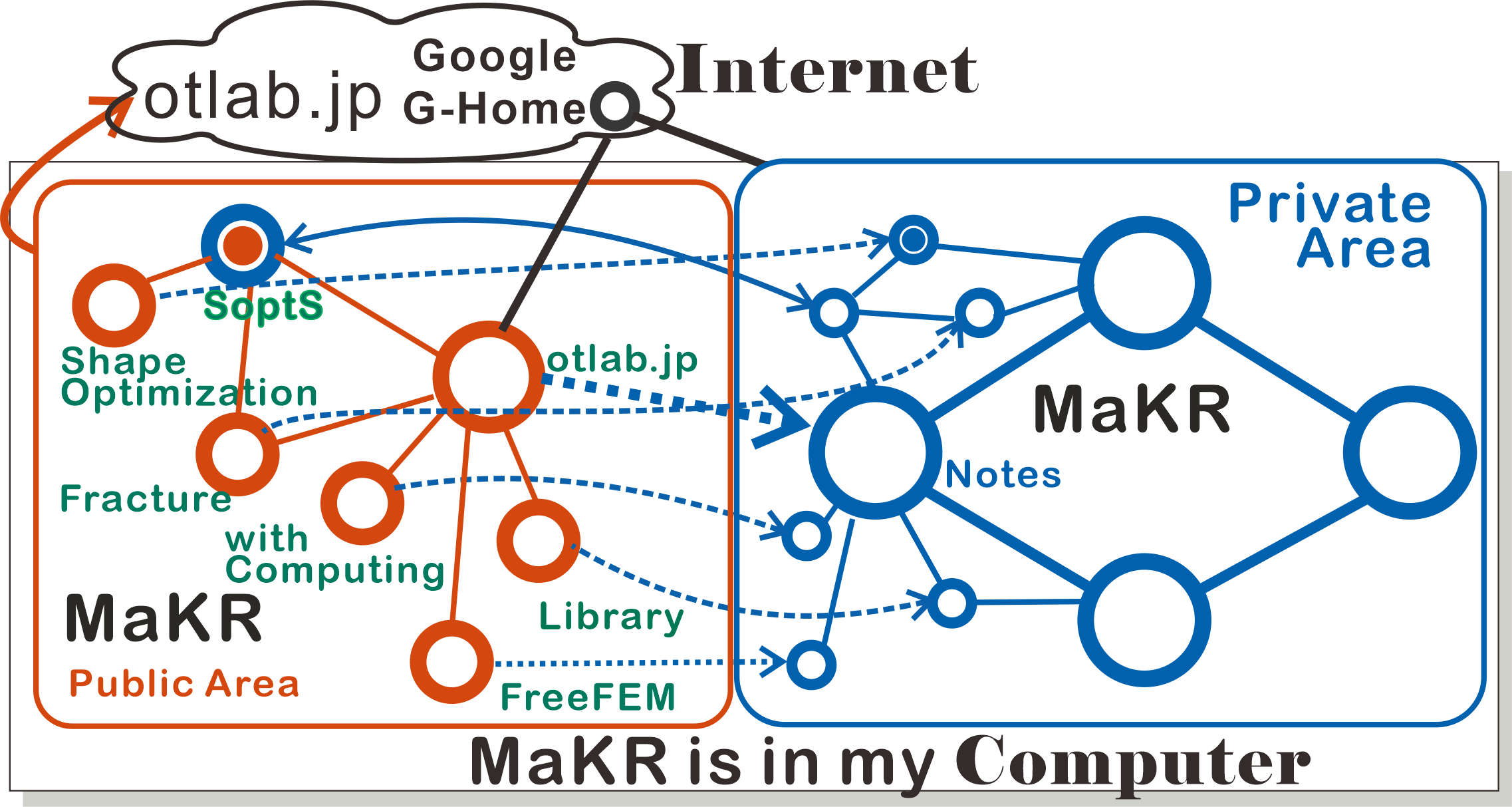
\end{eqnarray} Information about the page: The current position is painted circle in the diagram below. Blue is the main MaKR and orange is a duplicate for MaKR's public use, where dashed line means the connection to the private area The dashed lines are only connections to main MaKR.